| Su | Mo | Tu | We | Th | Fr | Sa |
|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | 7 |
| 8 | 9 | 10 | 11 | 12 | 13 | 14 |
| 15 | 16 | 17 | 18 | 19 | 20 | 21 |
| 22 | 23 | 24 | 25 | 26 | 27 | 28 |
| 29 | 30 | 31 |
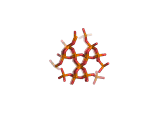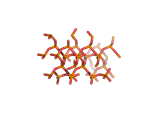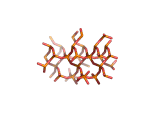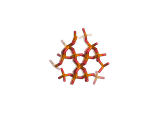
The properties of sets of atoms effectively control the modern world. For example, the atoms you see above form a small piece of silicon dioxide or SiO2. This model contains around 180 atoms and at its longest dimension is approximately 1.5 nanometers, or 1.5x10-9m, long.
Although this is a very small number of atoms, and a very small distance from one end of the group to the other, this size range is important to today's electronic devices. 1.5 nanometers marks the practical minimum limit to the thickness of SiO2 that can be used as an insulator in a component on an electronic chip. If you make the layer of SiO2 any smaller than this, too much current will flow through the supposedly insulating layer, and the device will overheat. So, SiO2 based insulators have a practical limit to their minimum dimensions.
The minimum dimensions of components on a chip determine the number of components on the chip. The more component that can be squeezed onto a chip, the more powerful the CPU, and the more responsive and impressive your computer or telephone. Additionally, in the energy conscious world of today, the smaller the device is, the lower its power consumption. So there are considerable incentives for miniaturization. However, all good things reach a limit, and now with a silicon dioxide thickness of 1.5 nanometers, the tiny leakage current which runs through the otherwise insulating SiO2 layer is a limiting factor which threatens to impede the inexorable advance in computing power.
The solution, so far, has been to employ not faster CPUs but more CPUs. Hence we have multi-core machines. However, there are a range of technological improvements on the horizon which will very likely accelerate the pace of development. There are new materials which can be made to adopt smaller dimensions than SiO2 and yet possess acceptable properties in devices. Additionally, it is possible that non-solid state electronic devices, based on organic molecules and their electronic properties will one day be able to take over from the silicon based semiconductors of today. However, whatever the technology you can be certain that the dimensions of these devices will be made ever smaller and their properties will be determined by small numbers of atoms, as in the model above.
The basic goal of the current administration's $75 billion mortgage relief plan is to keep up to 9 million Americans from losing their homes to foreclosure. That objective provides a wonderful opportunity to practice division with moderately large numbers. For example, how many dollars could go to each of those Americans threatened with losing their homes to foreclosure? The answer, if you do the math (as they say), is just $3,833.
One wishes it was actually more, but sadly, $3,833 is all each threatened homeowner would receive, on average.
Just as a revision of how one handles such a division, let's check! Seventy five billion is 75 thousand million. If you distribute 75 thousand million to 9 million people, each person receives 75 thousand divided by 9 (the millions cancel), or $8,333 dollars.
Of course, the current mortgage plan offers incentives to lenders to adjust mortgages. (Commercial entities can hardly be expected to change their terms without proper justification). So not all the money would actually go to the borrower. The government currently suggests paying loan service organizations $1,000 for each loan 'modification'. Additionally a bonus of $500 for each loan changed before borrowers turn 60 days delinquent would be awarded, and $1,000 a year for as much as three years, as long as the loan is serviced by the borrower. So the amount going to each borrower is reduced by $4,500, which is ear marked directly for the lender. (One cannot help but wonder which commercial concerns helped create the proposal.) However, this means that there is also a subtraction to be accounted for.
Fortunately, this subtraction can be straightforwardly handled too, the contribution to borrowers (on average) from the government will be less than $4,000. ($8,333-$4,500 = $3,833). No doubt there will be some overhead associated with administering the plan, because someone needs to decide who receives and who doesn't receive the assistance (there are many more than 9 million mortgages outstanding in the US), but let's ignore that additional cost.
The fact is that by the time you have completed the division and subtraction, less than $4,000 per borrower is left. You have to wonder whether this sum could possibly provide a meaningful impact on the problem. (Unless this is to be an annual government investment, of course).
In fact, the government already subsidizes mortgages, through tax relief on mortgage interest payments. So possibly a more efficient method of subsidizing mortgages might be to increase the amount that can be deducted against mortgage interest. Perhaps 150% of mortgage interest could be deducted against a borrower's taxable income. This would not require lender incentives (saving about half of the $75 billion) and would not require additional bureaucracy as the administration and collection system is already implemented. Additionally, this might encourage borrowers to maximize their income in order to maximize their tax relief, which would be good for the economy.